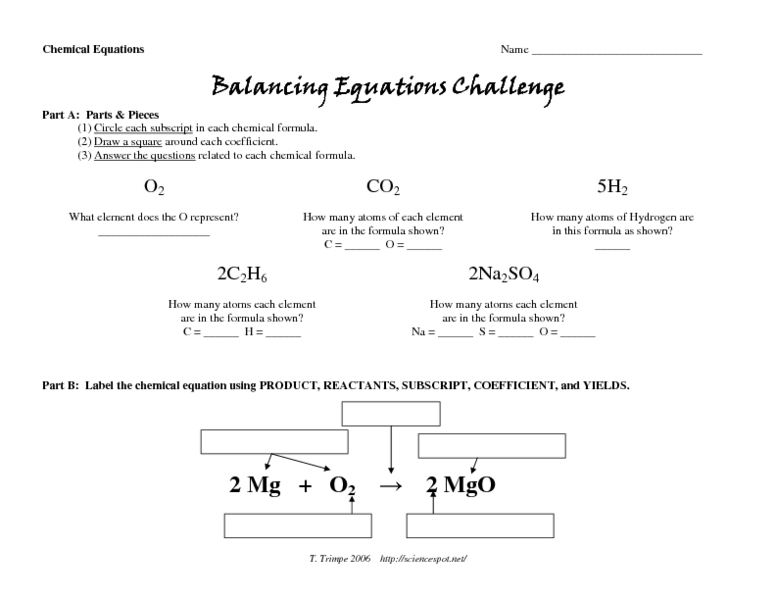
Challenge Questions Balance The Equations Below

Challenge questions balance the equations below, a multifaceted concept that intertwines problem-solving, analytical thinking, and engagement. This comprehensive guide delves into the intricacies of challenge questions, exploring their structures, techniques for balancing equations, and their applications in various fields.

Through a journey of discovery, we will uncover the benefits and drawbacks of different challenge question structures, delve into the rules and conventions for balancing equations, and explore strategies for solving even the most challenging equations.

Challenge Question Structures

Challenge questions are designed to test a student’s understanding of a concept and their ability to apply it to new situations. There are several different types of challenge question structures, each with its own benefits and drawbacks.

Open-ended questions

Allow students to demonstrate their understanding in a variety of ways.
Encourage critical thinking and problem-solving skills.
Can be difficult to grade objectively.

Multiple-choice questions

Provide a quick and easy way to assess student understanding.
Can be used to cover a wide range of content.
May not be as effective as open-ended questions for assessing higher-order thinking skills.

True/false questions

Can be used to assess student understanding of basic concepts.
Are easy to grade objectively.
May not be as effective as other types of questions for assessing higher-order thinking skills.

Balancing Equations

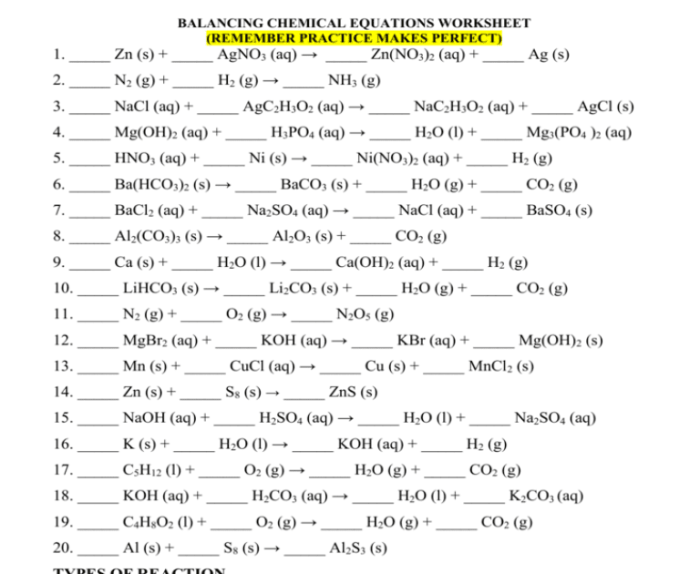
Balancing equations is a fundamental skill in chemistry. It involves adjusting the coefficients in front of the reactants and products in a chemical equation so that the number of atoms of each element is the same on both sides of the equation.

Rules for balancing equations

Coefficients can only be multiplied or divided by whole numbers.
The number of atoms of each element must be the same on both sides of the equation.
The coefficients in front of the reactants and products must be the smallest whole numbers possible.

Strategies for solving challenging equations

Start by balancing the most complex molecule first.
Use trial and error to find the coefficients that balance the equation.
Look for patterns in the equation that can help you find the coefficients.

Gamification and Engagement: Challenge Questions Balance The Equations Below

Challenge questions can be used to gamify the process of learning and make it more engaging for students. By turning equations into challenges, students can compete against each other or themselves to solve them in the fastest time or with the fewest mistakes.

Ways to gamify challenge questions

Create a leaderboard to track student progress.
Award points for correct answers and deduct points for incorrect answers.
Use timers to add an element of excitement.

Tips for creating engaging challenge questions

Make the questions challenging but not impossible.
Provide clear instructions and examples.
Use a variety of question types to keep students engaged.

Assessment and Feedback

Challenge questions can be used for assessment purposes to measure student understanding of a concept. They can also be used to provide feedback to students on their progress.

Methods for providing feedback on challenge questions

Provide written feedback on student answers.
Use automated feedback systems to provide immediate feedback.
Discuss student answers in class.

Role of challenge questions in identifying areas for improvement

Challenge questions can help identify areas where students need additional support.
They can also be used to track student progress over time.
By analyzing student answers to challenge questions, teachers can tailor their instruction to meet the needs of their students.

Real-World Applications

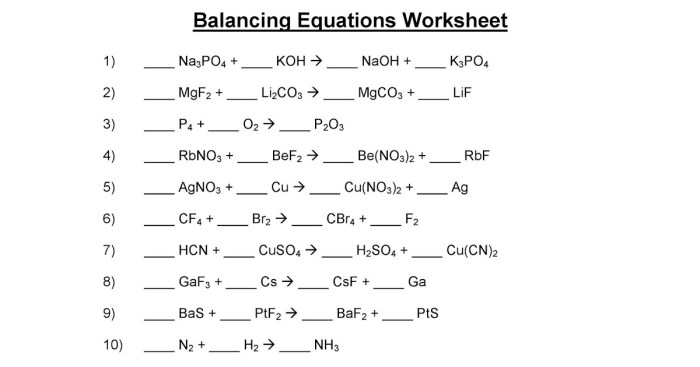
Challenge questions are not just limited to the classroom. They can be used in a variety of real-world contexts to solve problems and make decisions.

Examples of how challenge questions are used in real-world contexts

Engineers use challenge questions to design and build bridges, buildings, and other structures.
Doctors use challenge questions to diagnose and treat diseases.
Scientists use challenge questions to develop new technologies and products.

Benefits of using challenge questions in various industries and disciplines

Challenge questions can help professionals to think critically and solve problems.
They can also help professionals to stay up-to-date on the latest developments in their field.
By using challenge questions, professionals can improve their decision-making skills and become more effective in their roles.

Advanced Techniques

For more complex challenge questions, advanced techniques may be required to solve them. These techniques include:

Algebraic methods, Challenge questions balance the equations below

Using algebraic equations to represent the chemical equation.
Solving the equations to find the coefficients that balance the equation.

Geometric methods

Using geometric shapes to represent the chemical equation.
Manipulating the shapes to find the coefficients that balance the equation.

Resources for further exploration of advanced techniques

Balancing Chemical Equations: A Step-by-Step Guide: https://www.thoughtco.com/how-to-balance-chemical-equations-609268
Balancing Equations Using Algebra: https://www.khanacademy.org/science/chemistry/chemical-reactions/balancing-chemical-equations/a/balancing-chemical-equations-using-algebra
Balancing Equations Using Geometry: https://www.desmos.com/calculator/gfxtmx

glenn

Challenge Questions Balance The Equations Below